|
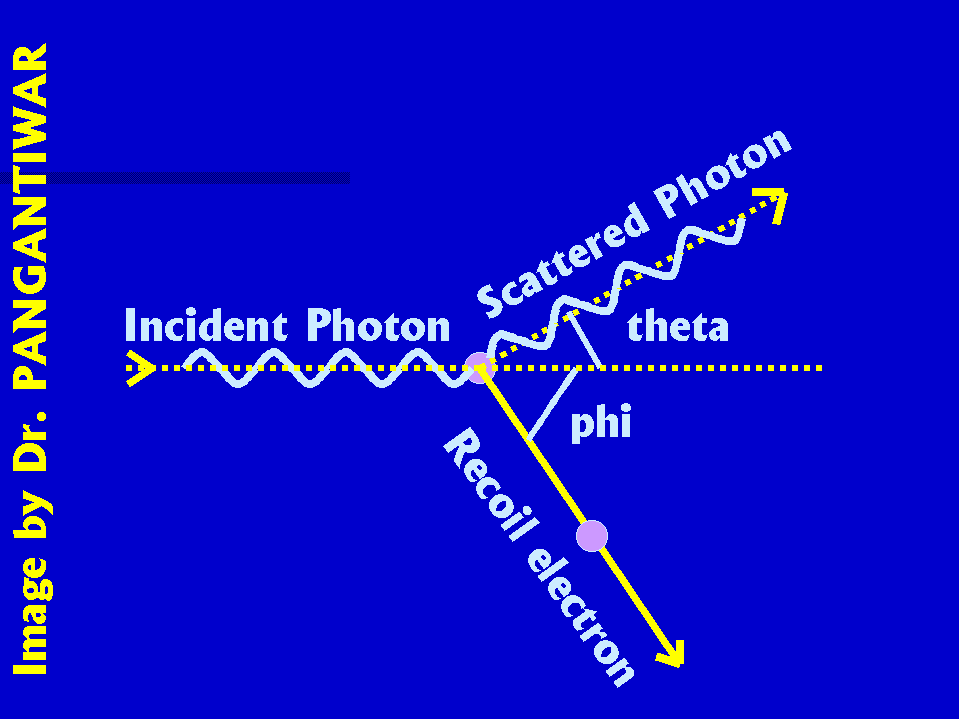
figure. Here (theta) is the scattering angle and (phi) is the recoil angle Before Collision (i) Energy of incident photon = h (freq) (ii) Momentum of incident photon = h (freq) / c (iii) Energy of the electron = m0 c2. Where m0 is rest mass of electron (iv) Momentum of electron at rest = 0 |
After Collision
(i) Energy of scattered photon = h (freq)'
(ii) Momentum of scattered photon = h (freq)' / c
(iii) Energy of the electron = m c2. Where m
is mass of electron moving with velocity v.
(iv) Momentum of recoil electron = mv, where v is the velocity of electron after collision.
and m = m0 / [square root of {1 - (v2 / c2)}]
According to the principle of conservation of energy
h (freq) + m0 c2 = h (freq)' + m c2 ...................(1)
Using the principle of conservation of momentum along and perpendicular to the direction of incidence, we get
h (freq) / c + 0 = [h (freq)' / c] cos(theta) + mv cos (phi) ...................(2)
0 + 0 = [h (freq)' / c] sin (theta) - mv sin(phi) ........................(3)
From equation (2), multiplying throughout by c, we get
h (freq) = h (freq)' cos(theta) + mvc cos(phi)
i.e. mvc cos(phi) = h (freq) - h (freq)' cos(theta)
Squaring, we get
m2v2c2 cos2(phi) = [h (freq) - h (freq)' cos(theta)]2.........(4)
From equation (3), multiplying throughout by c, we get
mvc sin(phi) = h (freq)' cos(theta)
Squaring, we get
m2v2c2 sin2(phi) = [h (freq)' sin(theta)]2.........(5)
Adding equation (4) with (5), we get
m2v2c2 cos2(phi) +
m2v2c2 sin2(phi) =
[h (freq) - h (freq)' cos(theta)]2 + [h (freq)' sin(theta)]2
i.e. m2v2c2 = h2 (freq)2
- 2 h (freq) h (freq)' cos(theta) + h2 (freq)'2 cos2(theta)
+ h2 (freq)'2 sin2(theta)
i.e. m2v2c2 = h2 [(freq)2
+ (freq)'2 - 2 (freq) (freq)' cos(theta)] ..........(6)
From equation (1), we get
mc2 = h [(freq) - (freq)'] + m0 c2
Squaring, we get
m2 c4 = h2 [(freq)2 - 2 (freq) (freq)' +
(freq)'2] + 2 h [(freq) - (freq)'] m0 c2
+ m02 c4 ..........(7)
Subtracting equation (6) from equation (7), we get
m2 c4 - m2v2c2 =
h2(freq)2 + h2(freq)'2 - 2 h (freq) h (freq)'
+ 2 h [(freq) - (freq)'] m0 c2 + m02 c4
- h2(freq)2 - h2(freq)'2 - 2 h (freq) h (freq)' cos(theta)
i.e. m2 c2 (c2 - v2) = - 2 h (freq) h (freq)'
+ 2 h [(freq) - (freq)'] m0 c2 + m02 c4
- 2 h (freq) h (freq)' cos(theta)
i.e. [m02 / (1 - v2 / c2)] c2 (c2 - v2) = - 2 h (freq) h (freq)'[1 - cos(theta)]
+ 2 h [(freq) - (freq)'] m0 c2 +
m02 c4
i.e. m02 c4 = - 2 h (freq) h (freq)'[1 - cos(theta)]
+ 2 h [(freq) - (freq)'] m0 c2 +
m02 c4
i.e. 2 h (freq) h (freq)'[1 - cos(theta)] = 2 h [(freq) - (freq)'] m0 c2
i.e. [h / (m0 c2)] [1 - cos(theta)] = [(freq) - (freq)'] / [(freq)(freq)']
i.e. [h / (m0 c2)] [1 - cos(theta)] = 1 / (freq)' - 1 / (freq)
i.e. [h / (m0 c2)] [1 - cos(theta)] = (lamda)' / c - (lamda) / c
i.e. Compton shift = (lamda)' - (lamda) = [h / (m0 c)] [1 - cos(theta)]
Thus we see that when photons collide elastically with loosely bound electrons of a Graphite sample,
the wavelength shift (Compton shift) of the scattered photons depends only on the scattering
angle and not on the incident wavelength.
==============
By :- Dr. A. W. Pangantiwar